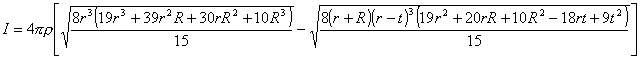

The moment of inertia of the shape is given by the equation
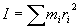
which is the sum of all the elemental particles masses multiplied by their distance from the rotational axis squared.
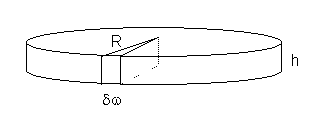
As the size of these particles tends to zero, it can be thought of as made up of small cubes with dimensions £Gw, £Gr and h,
So
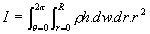
and
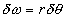
so
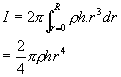
The mass of the whole disk is its volume multiplied by its density:
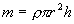
so the moment of inertia can be expressed as:
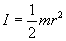
Consider the ‘corner?section of a cuboid as shown:
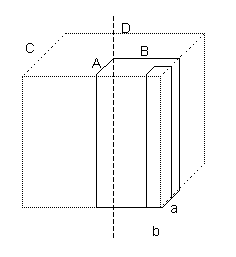
The ‘corner?shape with sides A and B is a quarter of the whole shape with sides C and D. The column with sides a and b is a very narrow column, whose sides tend to zero size.
As always, the moment of inertia of the whole shape is given by the equation
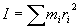
which is the sum of all the elemental particles masses multiplied by their distance from the rotational axis squared.
The mass of the narrow column is:
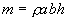
and its distance from the rotational axis, by Pythagoras, is
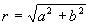
So

There are four of these corner blocks which go to make up a complete cuboid, hence for the complete cuboid:
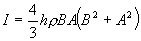
Given that A = C/2 and B = D/2?/p>
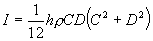
The mass of such a cuboid is given by
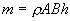
so
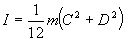
We can use the previous result to calculate an exact equation for a rectangular-section spoke. An approximate equation is often quoted for this shape for when the length is much greater than the width...
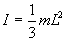
which can be used for any cross sectional shape, but let’s calculate the exact equation, then we can decide whether the approximation is valid or not depending on the circumstances.
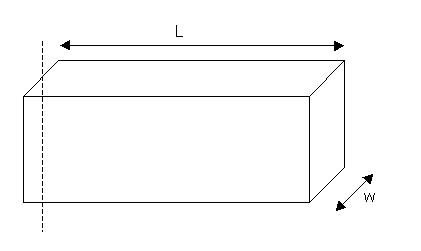
Using the result in section 2 for the corner block,
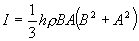
the moment of inertia of this wide spoke will be twice that of the corner block, since it constitutes two corner sections, so:
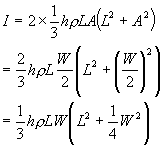
The mass of the spoke is
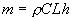
So

From this equation, we can see that if the width, W, is small compared with the length, L, the equation tends towards the common approximation.
If the spoke does not start at the axis of rotation, but a distance D from it, the moment of inertia can be calculated by subtracting the MOI of the ‘missing?section from the MOI of a spoke that does start at the axis,



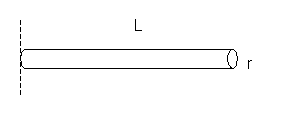
Calculating the exact moment of inertia for this shape is very difficult due to an impossible integration, and I haven’t figured out a way around that yet, so I can only present an approximation.
This approximation assumes that the radius of the spoke, r, is small compared with the length, L. Then we can assume that all the points on a disk cross section of the spoke are the same distance away from the rotational axis.
The moment of inertia is
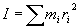
for each elemental particle. We will take a slice disk through the spoke of thickness ?i>dw?as the elemental particle, which will have a mass
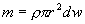
so
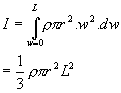
The mass of the spoke is

so
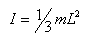
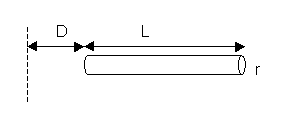
If the spoke does not start at the rotational axis, but a distance ‘D?from it, then

The mass of the spoke is

so
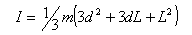

This shape is related to the cylinder, and the equation for moment of inertia can be found in the same manner as the cylinder, but by integrating from the inner radius to the outer instead of from 0:
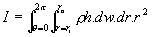
and
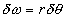
so

It can also be found by subtracting the moment of inertia of the ‘missing? inner cylinder from the outer cylinder:

This shape can be dealt with in two ways. First it can be done similarly to the solid block, but the lower limit of integration will not be zero. Secondly, and more easily, the moment of inertia can be calculated for the outer solid block, and then the moment of inertia of the missing inner block can be subtracted from it. This is the way we will do it.
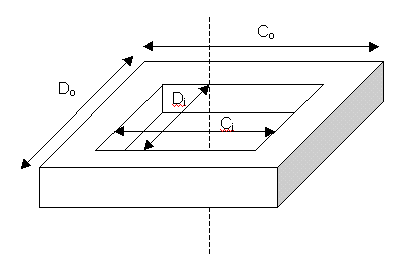
The equation for the outer shape, from before, is
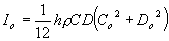
and the equation for the inner hollow would be

so the moment of inertia of the ring must be
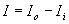
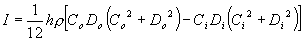
The moment of inertia is given by the equation
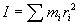
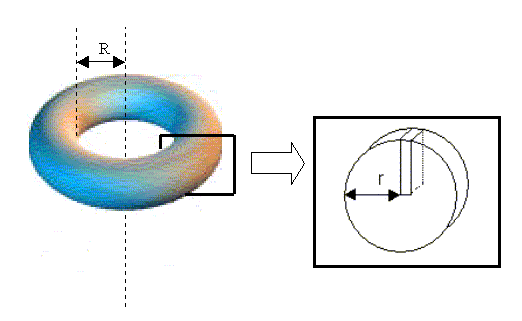
First imagine the torus made up of small disks as shown in the diagram above. Each of these disks is further made up of cuboid blocks as shown. Each block has a volume
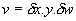
where £Gx is the width of the block (along the same direction as ‘r?, y is the height of the cuboid, and £Gw is the thickness of the circular disk, and therefore also the thickness of the cuboid. So given the density is £l, this cuboid has a mass

The height of the block, y, is given by the equation for a circle:
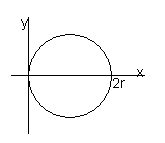
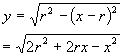
We can sum these masses up, multiplied by their distance from the rotational axis to get the moment of inertia. Of course we must sum them for the upper and lower semicircles, so we must multiply by 2.
For one such disk, the sum of the masses of the blocks and their distance from the rotational axis is given by the equation:
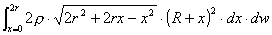
where (R + x) is the distance from the rotational axis.
The width of the circular disk, dw, depends on the distance from the central axis, and is given by the radius at that point times the angle. The radius at that point is (R + x), so:

These disks must then be summed around the whole circumference of the torus (i.e. from £c = 0 to £c = 360?/font> = 2£k), to give the total moment of inertia:
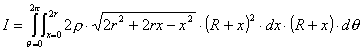
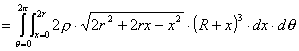
Let’s work on single sections at a time. First, the inner integral. This is rather difficult to do, and so I’ll yield to using Mathematica online, a superb tool available at http://integrals.wolfram.com/index.en.cgi. Just type in the equation and click on "Do It!".
Mathematica gives us the following answer for this inner integral:

You can see now why I never attempted to integrate it by hand! This rapidly simplifies as the limits x=0 and x=2r are inserted however:
When x=2r, this equation simplifies to:
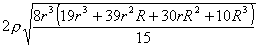
And when x=0, it becomes 0. So the whole inner integration result is just the first result (x=2r).
Now the outer integration. This is easy because there is no variable £c in the equation, so it becomes just 2 £k.
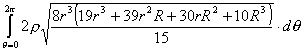
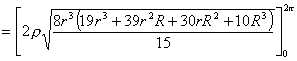

The moment of inertia of a hollow torus can be calculated simply using the equation derived for the solid torus. It is simply the MOI of an equivalent solid torus minus the MOI of the 'missing' hollow part.
Let r be the outer radius of the torus cross section, and R be the inner radius of the torus itself - the same as in the example above. We can imagine an 'air' torus, which is the gap inside the hollow torus. If the thickness of the material used to build the torus is t, then the radius of a cross section of the air torus is:
ra = r - t, and the inner radius of the air torus itself is Ra = R + t.
The MOI of the equivalent solid torus, from the last section, is:

The MOI of the air torus is:
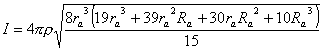
Note that the density for this air torus equation must still be that of the material used, since we are subtracting the MOI of the 'gap' created when a solid torus becomes a hollow one. So the MOI of the hollow torus is the MOI of the solid one minus the MOI of the gap:
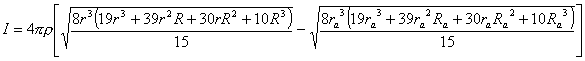
Replacing ra and Ra results in an equation in terms of the radius of the torus cross section, the radius of the torus itself, and the thickness and density of the material used: